Question
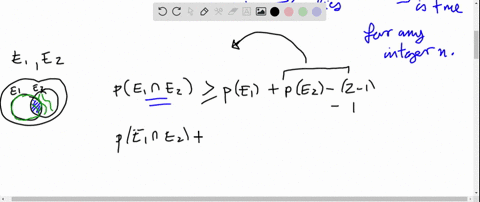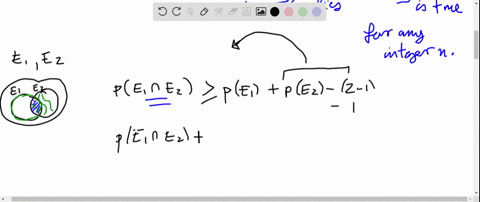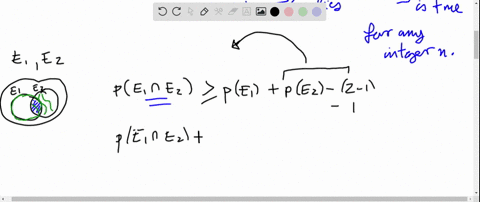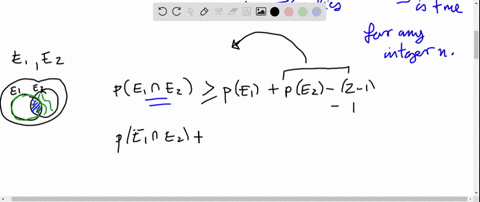
Use mathematical induction to prove the following generalization of Bonferroni's Inequality: $$ \begin{aligned} p\left(E_{1} \cap F_{i}\right.&\left.\cap \cdots \cap E_{n}\right) \\ & \geq p\left(E_{1}\right)+p\left(E_{2}\right)+\cdots+p\left(E_{a}\right)-(n-1) \end{aligned} $$ where $k_{1}, E_{2}, \ldots, E_{x}$ are $n$ events.
Use mathematical induction to prove the following generalization of Bonferroni's Inequality:Show more…
$$
\begin{aligned}
p\left(E_{1} \cap F_{i}\right.&\left.\cap \cdots \cap E_{n}\right) \\
& \geq p\left(E_{1}\right)+p\left(E_{2}\right)+\cdots+p\left(E_{a}\right)-(n-1)
\end{aligned}
$$
where $k_{1}, E_{2}, \ldots, E_{x}$ are $n$ events.
Chapter 5, Problem 14

Instant Answer
Step 1
The inequality becomes: $$ p(E_1) \geq p(E_1) + 0 $$ which is true. Show more…
Show all steps



Mengchun Cai and 52 other
Intro Stats / AP Statistics educators are ready to help you.
Ask a new question